Author: George Gamow
Publisher: Mentor (8th printing) 1959
One smart professor I know told me that he read this book when he was in
the 3rd grade. The author was a friend of fellow Hungarian mathematician John Von
Neumann. Neumann’s young daughter Marina—perhaps, she was in 3rd grade at the
time—told the author that she knows everything better than her
famous father does, except, of course, mathematics, which she
knows only equally well. So Gamow asked Marina to read the manuscripts of this book. After reading several chapters she told him that there are numerous things in the book that she could not understand. Then only the author realized that this book is not for
children as he originally intended it to be.
George Gamow is a famous physicist who predicted the big bang in
1940s. His graduate student Ralph Alpher did the calculations as part
of his PhD desertion. Gamow being facetious, included the name of Hans Bethe (The
Nobel Price winner for predicting the stelar nucleosynthesis) as one of the
authors of the article so that the names of the authors mimic the first
three letters of the Greek alphabet; alpha, beta, gamma: Alpher, Bethe,
Gamow. This paper is now known as the “alphabetical article”.
The book is divided in to four parts:
(1) Playing with numbers,
(2) Space, Time, and Einstein,
(3) Microcosmos, and
(4) Macrocosmos.
(1) Playing with numbers,
(2) Space, Time, and Einstein,
(3) Microcosmos, and
(4) Macrocosmos.
I was reading a book titled “Your Inner Fish” at the same time and it was
very interesting to see how science evolved from cutting edge science of
the 40s that Gamow writes in this book to the present. (The last chapter of part 3 is “The Riddle of Life”.)
I would like to include a story from the first chapter titled “Big
Numbers” (almost) in verbatim here.
In the great temple at Benares beneath the dome which marks the center of
the world, rests a brass plate in which are fixed three diamond needles,
each a cubit high (a cubit is about 51 centimeters) and as thick as a body
of a bee. On one of these
needles, at the creation, God placed sixty-four discs of pure gold, the
largest disc resting on the brass plate and the others getting smaller and
smaller up to the first one. This is the tower of Brahma. Day and night unceasingly, the priest on duty transfers discs from one
diamond needle to another, according to a fixed immaculate laws of Brahma,
which require that the priest must move only one disc at a time, and he
must place discs on needles so that there never is a smaller disc below a
larger one. When all
sixty-four discs shall have been thus transferred from the needle on
which, at the creation, God placed them, to one of the other needles,
tower, temple, and Brahmans alike will crumble into dust, and with a
thunder clap the world will vanish.
Assuming it takes a minute to transfer a disc from one needle to another,
how long does it take to finish this task? If we assume this time period is a culpa, then how long is a culpa in
years?
It was a delightful read and I highly recommend this book for everyone,
including the 3rd graders.
______
That was the end of my original note. The following sub-note is a result of a conversation I had with a friend who read this note.
______
That was the end of my original note. The following sub-note is a result of a conversation I had with a friend who read this note.
The answer to the above question is \(2^{64}-1\) minutes. (A mathematical
explanation is given at the end of this note.)
There are \(2^{64}-1\) minutes in a culpa. 60 minutes is an hour, 24
hours is a day, and there are 365 days in a year. So, you have to divide
\(2^{64}-1\) by \(60\times24\times365\) to get the answer in years. I
would like to stick to mental calculations as you have (no doubt)
done.
\(60 < 64 = 2^6\)
\(24 < 32 = 2^5\)
\(365 < 512 = 2^9\)
That is, \(60\times24\times365 < 2^{6+5+9} = 2^{20}\).
Therefore, as a ballpark figure, a කල්ප (culpa) is more than \(2^{44}\) years.
\(2^{44} = (2^4)\times(2^{40})\)
\(2^{10} = 1024\) which is approximately equal to \(1000 = 10^3\).
\(60 < 64 = 2^6\)
\(24 < 32 = 2^5\)
\(365 < 512 = 2^9\)
That is, \(60\times24\times365 < 2^{6+5+9} = 2^{20}\).
Therefore, as a ballpark figure, a කල්ප (culpa) is more than \(2^{44}\) years.
\(2^{44} = (2^4)\times(2^{40})\)
\(2^{10} = 1024\) which is approximately equal to \(1000 = 10^3\).
Therefore, a කල්ප
is approximately \(16\times10^{12}\) years.
That is, a කල්ප
as a ballpark figure is sixteen trillion years.
To give a new perspective, the universe is about 15 billion years old.
The sun and the planetary system is about 5 billion years old. In
another 5 billion years the sun will become a red giant and will absorb
the earth orbit. Therefore, whether we like it or not, there will be no
earth when the universe is 20 billion year old. Assuming this කල්ප
started 15 billion years ago, there will be 15 trillion, 980 billion
years left in this කල්ප
after the end of earth. So, those Brahmins in the great temple at
Benares will not finish the job, but all 8th graders know the
answer.
(1) The following is from “100 billion Suns—The Birth, Life, and Death of Stars” by Rudolf
Kippenhahn
The Sun creates energy by burning hydrogen and converting hydrogen to
helium. The Sun had enough hydrogen to last about 10 billion years. When
the Sun was formed 4.5 billion years ago it was about 30% dimmer than at
present. It is half-way through a process of shifting from a mode where
hydrogen is burned in a kernel at its center to a mode where hydrogen will
be burned in a spherical shell wrapped around an intensely hot, very
dense, but quite inert, helium core.
Once it makes the transition from core burning to shell burning, it will
be entering its twilight years. As the helium core grows, so does the
hydrogen-burning shell above it. The sun ball will continue to grow and in
about 7 billion years the sun will have grown to about one hundred times
its present size and its luminosity will be 2000 times as great. The
surface temperature will be about 4000 degrees centigrade, 1800 degrees
below its present level. The oceans of the Earth will have long since
evaporated; lead melts in the intensity of Sun's light. A gigantic red sun
spreading across more than half the sky shines down on an Earth devoid of
all life.
The scientific estimate is that the oceans will evaporate in about 850
million years and the surface temperature of Earth will be about 1600
degrees centigrade in about 1.15 billion years.
(2) The following interesting description is from the Buddhist Anguttara Nikaya (Numerical Discourses) VII (62):
(2) The following interesting description is from the Buddhist Anguttara Nikaya (Numerical Discourses) VII (62):
“After many many more years, it does not rain; and while it rains not,
all seedlings and vegetation, all plants, grasses, and trees dry up,
wither away and cease to be. There comes a season, at vast intervals in
the lapse of time, when a second sun appears. After the appearance of the
second sun, the brooks and ponds dry up, vanish away and cease to be.
There comes a season, at vast intervals in the lapse of time, when a third
sun appears; and thereupon the great rivers vanish away and cease to be.
At length, after another vast period, a fourth sun appears, and thereupon
the great lakes dry up, vanish away, and cease to be. After another long
lapse, a fifth sun appears, the great oceans dry up, vanish away, and
cease to be. After another lapse of time, a sixth sun appears; whereupon
this great earth reeks and fumes and send forth clouds of smoke. After a
last vast interval, a seventh sun appears, and then, this great earth
flares and blazes, and becomes one mass of flame.''
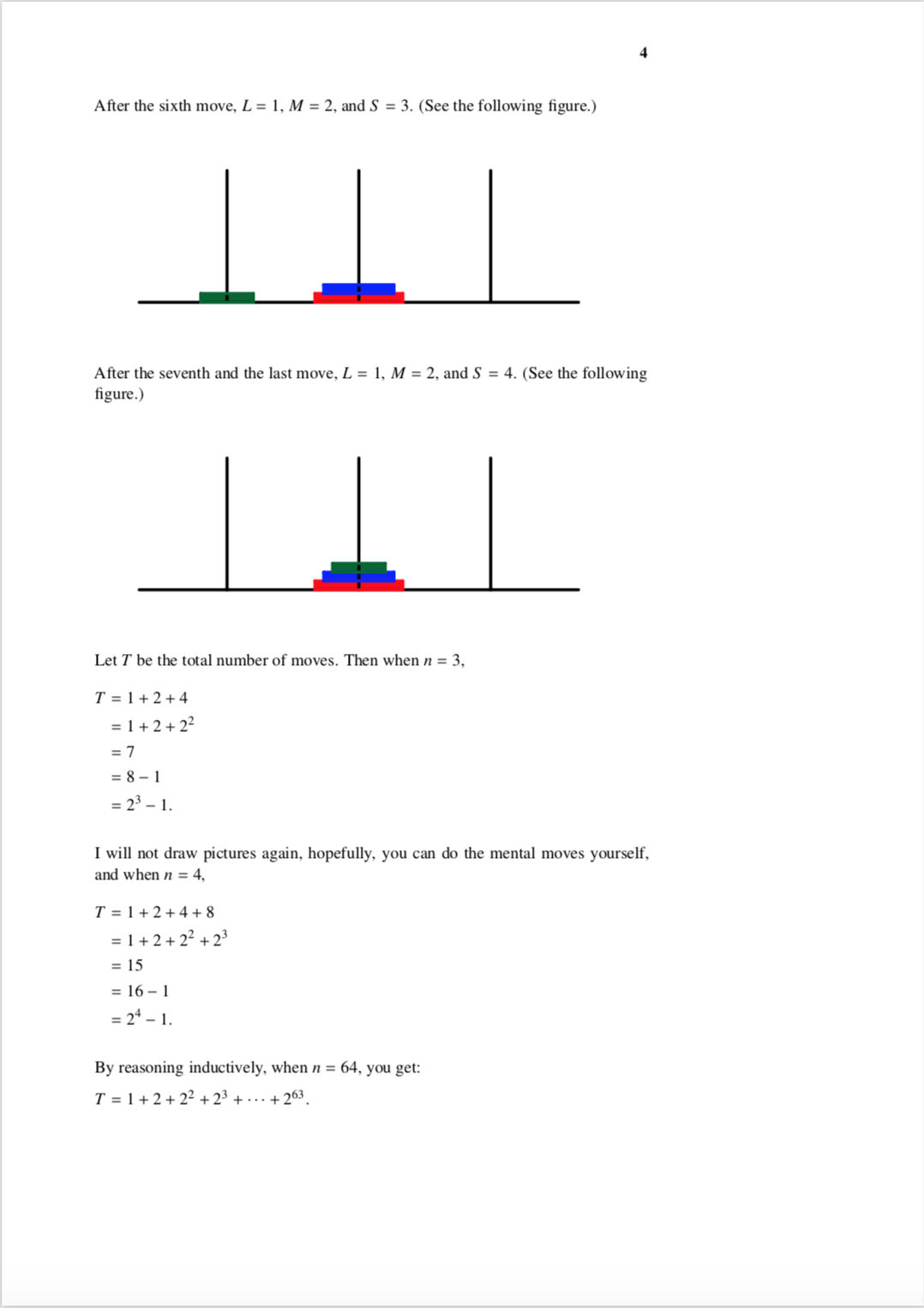
Mathematical Explanation:





No comments:
Post a Comment